The Poisson’s ratio of concrete varies 0.1 to 0.2.
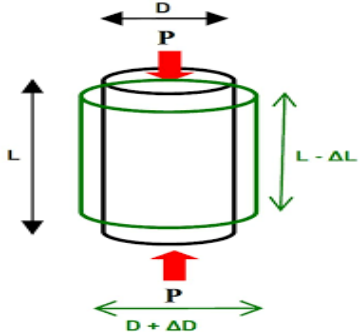
Poisson’s ratio (ν) is a measure of the ratio of lateral strain to longitudinal strain when a material is subjected to an axial load.
Table of Contents
Poisson’s Ratio Of Concrete
When a concrete specimen is subjected to axial loads the ratio of transverse strain to the longitudinal strain in concrete is termed Poisson’s ratio.
The concrete volume reduction under the compression load produces longitudinal and vertical strain. Crushing small aggregate sizes due to exerted loads causes volume reduction of concrete specimens. For determining the stress and deflection properties of structures such as beams, plates, and shells the Poisson’s ratio of concrete is taken as a constant.
Poisson’s ratio can be resolved progressively from ultrasonic pulse velocity from the fundamental resonant frequency of longitudinal vibration of the concrete beam.
Poisson’s Ratio Formula
For concrete, Poisson’s ratio can be calculated using the following formula:
Poisson’s ratio = (lateral strain) / (longitudinal strain)
Where:
- Lateral strain is the strain perpendicular to the direction of the applied load
- Longitudinal strain is the strain parallel to the direction of the applied load
Note: Poisson’s ratio of other materials also calculated by same formula.
Characteristics Of Concrete’s Poisson’s Ratio:
- Based upon the type of specimen (dry, saturated, or wet), and loading conditions the value of concrete Poisson’s ratio is possible to vary.
- Comparatively, Poisson’s ratio of concrete under the dynamic loads is greater than that of concrete upon which the static loads are applied.
- Under dynamic loads, the concrete Poissons ratio varies between 0.20 to 0.25.
- Generally, it varies between and ranges from 0.1 for high strength concrete to 0.2 for low strength concrete.
- The most common value of concrete Poisson’s ratio is taken as 0.2, for the design of concrete structures.
- Poisson’s ratio of concrete is constant up to about 70% of the strength.
- From the static modulus test, it can easily be computed.
- From ultrasonic pulse velocity, Poisson’s ratio can be determined dynamically and from the fundamental resonant frequency of longitudinal vibration of the concrete beam.
Importance Of Poisson’s Ratio Of Concrete
Poisson’s ratio influences the degree of shrinkage that concrete experiences during curing, resulting in cracks formation when there is higher lateral expansion.
The shear resistance of concrete is affected by Poisson’s ratio. A lower Poisson’s ratio increases shear resistance, which is crucial for designing structural elements such as beams and columns.
The elastic modulus of concrete is related to Poisson’s ratio, which impacts the stiffness of concrete. The elastic modulus is critical for designing concrete structures as it influences the degree of deflection under load.
Poisson’s ratio can also affect the formation and propagation of cracks in concrete. A lower Poisson’s ratio can result in cracks that are more perpendicular to the applied load, while a higher Poisson’s ratio can lead to cracks that are more diagonal.
Faqs
The Poisson’s ratio of concrete typically ranges from 0.1 to 0.2, with higher values indicating a higher degree of deformation in the lateral direction when the concrete is subjected to axial stress.
Yes, Poisson’s ratio of concrete can be altered by changing the properties of the concrete mix. For example, adding fibers or polymers to the mix can change the mechanical properties of the concrete, including Poisson’s ratio.
The Poisson’s ratio of concrete can affect the behavior of concrete structures under different loading conditions, such as compression, tension, and bending. Designers use the value of Poisson’s ratio to calculate the modulus of elasticity, shear modulus, and other mechanical properties, which are important for ensuring the safety and durability of the structure. It is also used to determine the amount of reinforcement needed in the structure to resist cracking or failure.
