Euler’s Theory of Column Buckling

Euler’s theory of column buckling is used to calculate the critical buckling load or the crippling load of a vertical strut or column.

Assumptions of Euler’s Theory of Column Buckling

Euler’s theory is based on some assumptions as given below.

1. Initially, the column is perfectly straight, homogeneous, isotropic, and obeys the hook’s law.
2. The cross-section is uniform throughout the length of the column. 
3. An axial load passes through the centroid of the section.
4. The stresses induced in the column are within the elastic limit.
5. The self-weight of the column is negligible.
6. Failure in the column occurs due to buckling only.
7. Column length is very large compared to its cross-sectional dimensions.
8. Both ends of the column are frictionless.
9. The shortening of the column because of axial compression is negligible.

Formula to Calculate Critical Buckling Load

Euler’s crippling load is given by the following formula.

P_e = EI(PI/KL) ^2 

where,

P_e= crippling load,

E: Young’s Modulus of elasticity,

I: Moment of inertia ( MOI )

L: Length of the slender column,

K: Effective length factor

This formula to calculates column buckling load was given by the Swiss mathematician Leonhard Euler in 1757. 

The effective length factor depends on various end conditions as given in the below image.

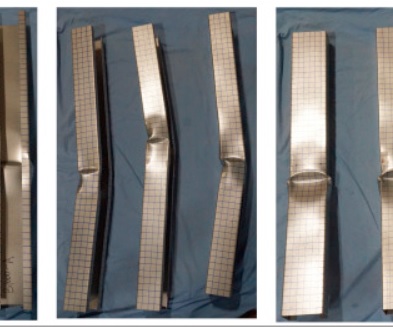
Here are some real-world photos of buckled columns.