Stirling cycle is a thermodynamic cycle that included two isothermal and two isochoric measures. Heat dismissal and heat expansion happen at a constant temperature.
The ideal Otto cycles and Diesel cycles are not absolutely reversible since they include heat move through a limited temperature contrast during the irreversible isochoric/isobaric heat-expansion and heat-rejection processes. The irreversibility delivers the thermal effectiveness of these cycles not exactly that of a Carnot motor working inside similar restrictions of temperature.
Another cycle that highlights isothermal heat expansion and heat dismissal measures is the Stirling cycle, which is a changed adaptation of the Carnot cycle in which the two isentropic cycles included in the Carnot cycle are supplanted by two steady volume recovery measures.
Working Principal
The framework includes two cylinders for a chamber with a regenerator in the center. At first
the left chamber houses the whole working liquid (a gas) at high strain and high
temperature.
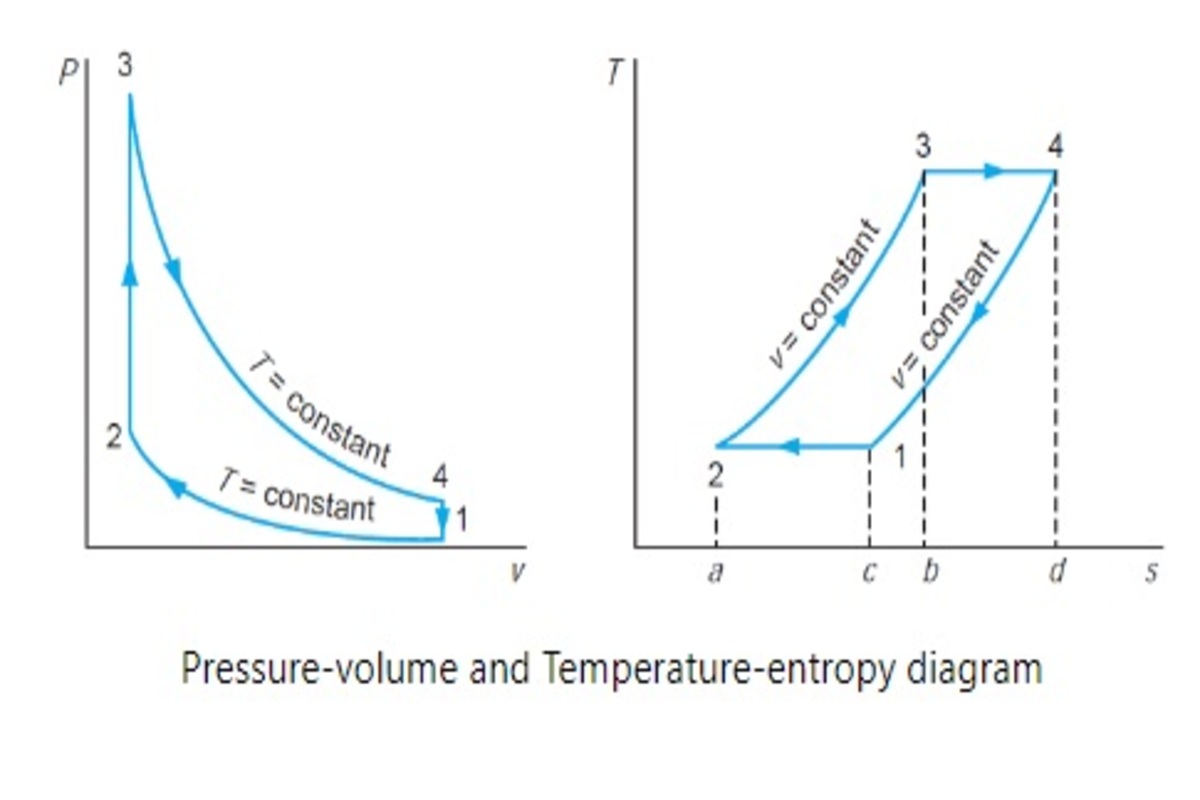
Where,
1-2: Isothermal compression
2-3: Constant volume cooling
3-4: Isothermal expansion
4-1: Contact volume heating
From the p-V and T-s diagram of the Stirling cycle, it is very clear that the amount of heat addition and heat rejection during constant volume is the same.

Heat supplied = Work done during isothermal expansion

Heat rejected by the air during isothermal compression
Work done = heat supplied – heat rejected

Thermal efficiency can be given by the equation

